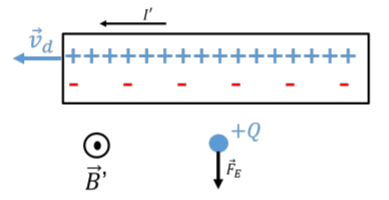
What happens with the number of protons inside a wire in movement?

The density $rho$ of the protons increase with the factor $gamma$, but the length $L$ of the wire decreases with the same factor. So the total number of protons will be unchanged: $(gammarho)*(L/gamma) = rho L$.

In the frame moving with the current, the hyperplane of simultaneity (aka 'now') is tilted to the lab's future (past) in the downstream (upstream) direction.

OR: If you are in the lab frame, every time an electron leaves the wire (event B) at the downstream end, one enters to replace it at the upstream end (event A), thereby maintaining neutrality. A and B are simultaneous in the lab.

In the current frame, B occurs before A.

But if the density of protons is higher, then the number of protons must be higher too, so changing the frame of reference is also creating new protons? How can we explain the higher density without adding extra protons?

Here is an electric circuit according to an observer standing still: O Here is the same electric circuit according to an observer moving past it: 0

Let's say the observers are at the center of the O or 0. Now we can see that the moving observer sees protons packed together when he looks up or down.

To clarify what "up" means, here is a picture of an observer standing up like a stick: |

At his left and his right he sees protons being extra close to himself. That has no effect though, because the electric fields of protons have changed shape too. Still standing proton's electric field: O Moving protons electric field: 0

In the usual presentation of this derivation, the wire is infinite in length, which means that the total number of protons in the wire is infinite. Increasing the proton density in the wire is not a problem in this case, because the "total number" of protons is "the same". No proton creation is necessary, because an infinite wire with 1 proton/unit length and an infinite wire with 2 protons/unit length both have a countably infinite number of protons.

This is very similar to the discussions about infinity that arise in "Hilbert's Hotel": in a hotel with rooms labeled 1,2,..., you can accomodate any additional number of guests you want without having to create additional rooms.

Normally this idea is presented in the case of a wire that is infinitely long. That gives the problem a translational symmetry and makes everything simpler. For an infinite wire, the number of protons is infinite. If you change to a different frame, the number is still infinite, but with a different density. This is similar to Hilbert's hotel: https://en.wikipedia.org/wiki/Hilbert%27s_paradox_of_the_Grand_Hotel